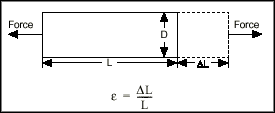
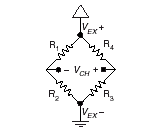Basic Elements of a Pneumatic System

A – Compressor: a pump which compresses air, raising it to a higher pressure, and delivers it to the pneumatic system (sometimes, can also be used to generate a vacuum).B – Check valve: one-way valve that allows pressurized air to enter the pneumatic system, but prevents backflow (and loss of pressure) into the compressor when it is stopped.C – Accumulator: stores compressed air, preventing surges in pressure and relieving the duty cycle of the compressor.D – Directional valve: controls the flow of pressurized air from the source to the selected port. Some valves permit free exhaust from the
Read more »
Labels:

What is Mechatronics?

Back in the early 1970s, Japanese industry coined the word Mechatronics in relation to the development of the world's first industrial robots. Robotics has since come to be regarded as a generic term, and yet it forms only a subset of Mechatronics, which still lacks recognition in some quarters as an indigenous term in our technological vocabulary. 'Mechatronics ' is a natural choice for explaining a process that seeks, from the outset, to generate definitive engineering system solutions, which are inextricably bound by those integrating technologies associated with the inveterate mechanical,
Read more »
Labels:

Some Common Pneumatic Circuits

Controlling Cylinder Speed Five Ported Four Way Description: 4-Way 4 Flow Directions 2 - Position Actuated/at rest Normally Passing Classification Not Applicable Push Button, Spring Return Operator Type 5 Ports 1, 2, 3, 4, and 5 Each cylinder port has its own exhaust Standard labels apply --- ISO standard shown above, may also be: Sandwich Speed Control NOTE: Dotted line indicates an assembly --- a modular combination of devices; in this case a valve and a speed control sandwich. Metering devices are needle valves and serve to restrict only
Read more »
Labels:

Strain Gauge Configuration Types

Wheatstone Bridges and Strain Gauges All strain-gauge configurations are based on the concept of a Wheatstone bridge. A Wheatstone bridge is a network of four resistive legs. One or more of these legs can be active sensing elements. Figure 1-1 shows a Wheatstone bridge circuit diagram. Figure 1-1. Basic Wheatstone Bridge Circuit Diagram The Wheatstone bridge is the electrical equivalent of two parallel voltage divider circuits. R1 and R2 compose one voltage divider circuit, and R4 and R3 compose the second voltage divider circuit. The output of a Wheatstone bridge is measured between the
Read more »
Labels:

Strain and Strain Gauges

What Is Strain? Strain is the amount of deformation of a body due to an applied force. More specifically, strain (e) is defined as the fractional change in length, as shown in Figure 1 below. Figure 1. Definition of Strain Strain can be positive (tensile) or negative (compressive). Although dimensionless, strain is sometimes expressed in units such as in./in. or mm/mm. In practice, the magnitude of measured strain is very small. Therefore, strain is often expressed as microstrain (me), which is e x 10-6.When a bar is strained with a uniaxial force, as in Figure 1, a phenomenon known as Poi
Read more »
Labels: ,